MCQ Questions for Class 12 Maths Chapter 2 Inverse Trigonometric Functions with Answers
Question 1.
sin-1 (sin\(\frac{2π}{3}\)) =
(a) \(\frac{2π}{3}\)
(b) \(\frac{π}{6}\)
(c) \(\frac{4π}{3}\)
(d) \(\frac{π}{3}\)
Answer
Answer: (d) \(\frac{π}{3}\)
Question 2.
sin-1 (1 – x) – 2 sin-1 x = \(\frac{π}{2}\) then x = ?
(a) 0, \(\frac{1}{2}\)
(b) 1, \(\frac{1}{2}\)
(c) \(\frac{1}{2}\)
(d) 0
Answer
Answer: (d) 0
Question 3.
tan-1 √3 – sec-1(-2)
(a) π
(b) –\(\frac{π}{3}\), 0
(c) \(\frac{π}{3}\)
(d) \(\frac{2π}{3}\)
Answer
Answer: (b) –\(\frac{π}{3}\), 0
Question 4.
sin(sec-1 x + cosec-1x) =
(a) 1
(b) -1
(c) \(\frac{π}{2}\)
(d) \(\frac{π}{3}\)
Answer
Answer: (a) 1
Question 5.
2 tan-1 \(\frac{1}{3}\) + tan-1 \(\frac{1}{7}\) =
(a) tan-1 \(\frac{44}{29}\)
(b) \(\frac{π}{2}\)
(c) 0
(d) \(\frac{π}{4}\)
Answer
Answer: (d) \(\frac{π}{4}\)
Question 6.
The principle value of sin-1 \(\frac{√3}{2}\) is
(a) \(\frac{2π}{3}\)
(b) \(\frac{π}{6}\)
(c) \(\frac{π}{4}\)
(d) \(\frac{π}{3}\)
Answer
Answer: (d) \(\frac{π}{3}\)
Question 7.
The value of the expression tan-1(\(\frac{1}{2}\)cos-1\(\frac{2}{√5}\)) is
(a) 2 + √5
(b) √5 – 2
(c) \(\frac{√5+2}{4}\)
(d) √5 + 2
Answer
Answer: (d) √5 + 2
Question 8.
Simplified form of cos-1 (4x3 – 3x)
(a) 3 sin-1x
(b) 3 cos-1x
(c) π – 3 sin-1x
(d) None of these
Answer
Answer: (b) 3 cos-1x
Question 9.
The value of tan(tan-1 \(\frac{4}{5}\) + tan-1 \(\frac{2}{3}\)) is
(a) \(\frac{6}{17}\)
(b) \(\frac{7}{16}\)
(c) \(\frac{17}{6}\)
(d) None of these
Answer
Answer: (d) None of these
Question 10.
tan-1(\(\frac{x}{y}\)) – tan-1(\(\frac{x-y}{x+y}\)) is equal to
(a) \(\frac{π}{3}\)
(b) \(\frac{π}{4}\)
(c) \(\frac{π}{2}\)
(d) \(\frac{-3π}{4}\)
Answer
Answer: (b) \(\frac{π}{4}\)
Question 11.
The value of x for which sin |cot-1(1 – x)| = cos (tan-1 x) is
(a) \(\frac{2}{1}\)
(b) 1
(c) 0
(d) \(\frac{1}{2}\)
Answer
Answer: (d) \(\frac{1}{2}\)
Question 12.
Princal value of cos-1(\(\frac{-1}{√2}\))
(a) \(\frac{3π}{4}\)
(b) \(\frac{5π}{4}\)
(c) –\(\frac{π}{4}\)
(d) None of these
Answer
Answer: (a) \(\frac{3π}{4}\)
Question 13.
tan-1 √3 – sec-1 (-2) is equal to
(a) π
(b) –\(\frac{π}{3}\)
(c) \(\frac{π}{3}\)
(d) \(\frac{2π}{3}\)
Answer
Answer: (b) –\(\frac{π}{3}\)
Question 14.
If y = sec-1 x then
(a) 0 ≤ y ≤ π
(b) 0 ≤ y ≤ \(\frac{π}{2}\)
(c) –\(\frac{π}{2}\) < y < \(\frac{π}{2}\)
(d) None of these
Answer
Answer: (d) None of these
Question 15.
If x + \(\frac{1}{x}\) = 2 then the principal value of sin-1 x is x
(a) \(\frac{π}{4}\)
(b) \(\frac{π}{2}\)
(c) π
(d) \(\frac{3π}{2}\)
Answer
Answer: (d) \(\frac{3π}{2}\)
Question 16.
4 tan-1 \(\frac{1}{5}\) – tan-1 \(\frac{1}{239}\)
(a) π
(b) \(\frac{π}{2}\)
(c) \(\frac{π}{3}\)
(d) \(\frac{π}{4}\)
Answer
Answer: (d) \(\frac{π}{4}\)
Question 17.
The principle value of sin-1(sin\(\frac{2π}{3}\)) is
(a) \(\frac{2π}{3}\)
(b) \(\frac{π}{3}\)
(c) \(\frac{-π}{6}\)
(d) \(\frac{π}{6}\)
Answer
Answer: (b) \(\frac{π}{3}\)
Question 18.
The value of cos-1(\(\frac{1}{2}\)) + 2sin-1(\(\frac{1}{2}\)) is equal to
(a) \(\frac{π}{4}\)
(b) \(\frac{π}{6}\)
(c) \(\frac{2π}{3}\)
(d) \(\frac{5π}{6}\)
Answer
Answer: (b) \(\frac{π}{6}\)
Question 19.
Algebraic expression for sin (cot-1 x) is
(a) \(\frac{1}{1+x^2}\)
(b) \(\frac{1}{\sqrt{1+x^2}}\)
(c) \(\frac{x}{\sqrt{1+x^2}}\)
(d) None of these
Answer
Answer: (b) \(\frac{1}{\sqrt{1+x^2}}\)
Question 20.
If sin-1(\(\frac{2x}{1+x^2}\)) + sin-1\(\frac{2y}{1+y^2}\) = 2 tan-1 a then a is equal to
(a) \(\frac{x-y}{1+xy}\)
(b) \(\frac{y}{1+xy}\)
(c) \(\frac{y}{1-xy}\)
(d) \(\frac{x+y}{1-xy}\)
Answer
Answer: (d) \(\frac{x+y}{1-xy}\)
Question 21.
Princal value of tan-1 (-1) is
(a) \(\frac{π}{4}\)
(b) \(\frac{-π}{2}\)
(c) \(\frac{5π}{4}\)
(d) \(\frac{-π}{4}\)
Answer
Answer: (d) \(\frac{-π}{4}\)
Question 22.
tan-1(\(\frac{1}{4}\)) + tan-1(\(\frac{2}{9}\)) equal to
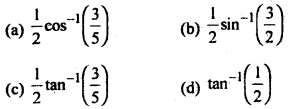
Answer
Answer: (d) tan-1(\(\frac{1}{2}\))
Question 23.
Principal value of sin-1(\(\frac{1}{√2}\))
(a) \(\frac{π}{4}\)
(b) \(\frac{3π}{4}\)
(c) \(\frac{5π}{4}\)
(d) None of these
Answer
Answer: (a) \(\frac{π}{4}\)
Question 24.
sin-1 x = y Then
(a) 0 ≤ y ≤ π
(b) –\(\frac{π}{2}\) ≤ y ≤ \(\frac{π}{2}\)
(c) 0 < y < π
(d) –\(\frac{π}{2}\) < y < –\(\frac{π}{2}\)
Answer
Answer: (b) –\(\frac{π}{2}\) ≤ y ≤ \(\frac{π}{2}\)
Question 25.
cos-1(cos\(\frac{7π}{6}\)) is equal to
(a) \(\frac{7π}{6}\)
(b) \(\frac{5π}{6}\)
(c) \(\frac{π}{3}\)
(d) \(\frac{π}{6}\)
Answer
Answer: (b) \(\frac{5π}{6}\)
Question 26.
sin[\(\frac{π}{3}\) – sin-1(-\(\frac{1}{2}\))] is equal to
(a) \(\frac{1}{2}\)
(b) \(\frac{1}{3}\)
(c) \(\frac{1}{4}\)
(d) 1
Answer
Answer: (d) 1
Question 27.
tan-1\(\frac{1}{2}\) + tan-1\(\frac{2}{11}\) = tan-1 a then a = ?
(a) \(\frac{1}{4}\)
(b) \(\frac{1}{2}\)
(c) \(\frac{3}{4}\)
(d) 1
Answer
Answer: (c) \(\frac{3}{4}\)
Question 28.
tan-1\(\frac{1}{2}\) + tan-1\(\frac{1}{3}\) =
(a) \(\frac{π}{4}\)
(b) \(\frac{π}{2}\)
(c) \(\frac{π}{3}\)
(d) π
Answer
Answer: (a) \(\frac{π}{4}\)
Question 29.
If sin-1 x + sin-1 y = \(\frac{2π}{3}\) then cos-1 x + cos-1 y =
(a) \(\frac{2π}{3}\)
(b) \(\frac{π}{4}\)
(c) \(\frac{π}{3}\)
(d) \(\frac{π}{2}\)
Answer
Answer: (c) \(\frac{π}{3}\)
Question 30.
The principal value of cosec-1 (-2) is
(a) –\(\frac{2π}{3}\)
(b) \(\frac{π}{6}\)
(c) \(\frac{2π}{3}\)
(d) –\(\frac{π}{6}\)
Answer
Answer: (d) –\(\frac{π}{6}\)
Question 31.
The domain of the following f(x) = \(\sqrt{sin^{-1}x}\) is
(a) [0, 1]
(b) [-1, 1]
(c) [-, 0]
(d) [0, 1]
Answer
Answer: (a) [0, 1]
Question 32.
Which of the following is the principal value branch of cos-1 x?
(a) [\(\frac{-π}{2}\), \(\frac{π}{2}\)]
(b) (0, π)
(c) (0, π)
(d) (0, π) – {\(\frac{π}{2}\)}
Answer
Answer: (c) (0, π)
Question 33.
Which of the following is the principal value branch of cosec-1 x?
(a) (\(\frac{-π}{2}\), \(\frac{π}{2}\))
(b) (0, π) – {\(\frac{π}{2}\)}
(c) [\(\frac{-π}{2}\), \(\frac{π}{2}\)]
(d) [\(\frac{-π}{2}\), \(\frac{π}{2}\)] – [0]
Answer
Answer: (d) [\(\frac{-π}{2}\), \(\frac{π}{2}\)] – [0]
Question 34.
If 3 tan-1 x + cot-1 x = π, then x equals
(a) 0
(b) 1
(c) -1
(d) \(\frac{1}{2}\)
Answer
Answer: (b) 1
Question 35.
The value of cos-1[cos(\(\frac{33π}{5}\))] is
(a) \(\frac{3π}{5}\)
(b) \(\frac{-3π}{5}\)
(c) \(\frac{π}{10}\)
(d) –\(\frac{-π}{10}\)
Answer
Answer: (a) \(\frac{3π}{5}\)
Question 36.
The domain of the function cos-1 (2x – 1) is
(a) [0, 1]
(b) [-1, 1]
(c) [-1, -1]
(d) [0, π]
Answer
Answer: (a) [0, 1]
Question 37.
The domain of the function defined by f (x) = sin-1 \(\sqrt{x-1}\) is
(a) [1, 2]
(b) [-1, 1]
(c) [0, 1]
(d) None of these
Answer
Answer: (a) [1, 2]
Question 38.
If cos(sin-1\(\frac{2}{5}\) + cos-1 x) = 0 then x is equal to
(a) \(\frac{1}{5}\)
(b) \(\frac{2}{5}\)
(c) 0
(d) 1
Answer
Answer: (b) \(\frac{2}{5}\)
Question 39.
The value of sin (2 tan-1 (.75)) is equal to
(a) .75
(b) 1.5
(c) .96
(d) sin 1.5
Answer
Answer: (c) .96
Question 40.
The value of cos-1 (cos\(\frac{3π}{2}\)) is equal to
(a) \(\frac{π}{2}\)
(b) \(\frac{3π}{2}\)
(c) \(\frac{5π}{2}\)
(d) –\(\frac{7π}{2}\)
Answer
Answer: (a) \(\frac{π}{2}\)
Question 41.
The value of expression 2 sec-1 2 + sin-1 (\(\frac{1}{2}\)) is
(a) \(\frac{π}{6}\)
(b) \(\frac{5π}{6}\)
(c) \(\frac{7π}{6}\)
(d) 1
Answer
Answer: (b) \(\frac{5π}{6}\)
Question 42.
If tan-1 x + tan-1 y = \(\frac{4π}{5}\) then cot-1 x + cot-1 y equals
(a) \(\frac{π}{5}\)
(b) \(\frac{2π}{5}\)
(c) \(\frac{3π}{5}\)
(d) π
Answer
Answer: (a) \(\frac{π}{5}\)
Question 43.
If sin-1(\(\frac{2a}{1+a^2}\)) + cos-1(\(\frac{1-a^2}{1+a^2}\)) = tan-1(\(\frac{2x}{1-x^2}\)) where a, x ∈ |0, 1| then the value of x is
(a) 0
(b) \(\frac{a}{2}\)
(c) a
(d) \(\frac{2a}{1-a^2}\)
Answer
Answer: (d) \(\frac{2a}{1-a^2}\)
Question 44.
The value of sin [cos-1(\(\frac{7}{25}\))] is
(a) \(\frac{25}{24}\)
(b) \(\frac{25}{7}\)
(c) \(\frac{24}{25}\)
(d) \(\frac{7}{24}\)
Answer
Answer: (c) \(\frac{24}{25}\)
Question 45.
If |x| ≤ 1, then 2 tan-1 x + sin-1(\(\frac{2x}{1+x^2}\)) is equal to
(a) 4 tan-1 x
(b) \(\frac{π}{2}\)
(c) 0
(d) π
Answer
Answer: (a) 4 tan-1 x
Question 46.
If cos-1 α + cos-1 β + cos-1 γ = 3π, then α(β + γ) + β (γ + α) + γ(α + β) equals
(a) 0
(b) 1
(c) 6
(d) 12
Answer
Answer: (c) 6
Question 47.
The number of real solution of the equation is
\(\sqrt{1+cos 2x}\) = √2 cos-1(cos x) in [\(\frac{π}{2}\), π] is
(a) 0
(b) 1
(c) 2
(d) None of these
Answer
Answer: (c) 2
Question 48.
If cos-1 x > sin-1 x, then
(a) \(\frac{1}{√2}\) < x ≤ 1
(b) 0 ≤ x < \(\frac{1}{√2}\)
(c) -1 ≤ x < \(\frac{1}{√2}\) (d) x > 0
Answer
Answer: (b) 0 ≤ x < \(\frac{1}{√2}\)
Question 49.
sin-1(\(\frac{-1}{2}\))
(a) \(\frac{π}{3}\)
(b) –\(\frac{π}{3}\)
(c) \(\frac{π}{6}\)
(d) –\(\frac{π}{6}\)
Answer
Answer: (d) –\(\frac{π}{6}\)
Question 50.
sec-1(\(\frac{-2}{√3}\))
(a) \(\frac{π}{6}\)
(b) \(\frac{π}{3}\)
(c) \(\frac{5π}{6}\)
(d) –\(\frac{2π}{3}\)
Answer
Answer: (c) \(\frac{5π}{6}\)
Question 51.
cos-1(\(\frac{1}{2}\))
(a) –\(\frac{π}{3}\)
(b) \(\frac{π}{3}\)
(c) \(\frac{π}{2}\)
(d) \(\frac{2π}{3}\)
Answer
Answer: (b) \(\frac{π}{3}\)
Question 52.
cosec-1(\(\frac{-2}{√3}\))
(a) –\(\frac{π}{3}\)
(b) \(\frac{π}{3}\)
(c) \(\frac{π}{2}\)
(d) –\(\frac{π}{2}\)
Answer
Answer: (a) –\(\frac{π}{3}\)
Question 53.
cot-1(1)
(a) \(\frac{π}{3}\)
(b) \(\frac{π}{4}\)
(c) \(\frac{π}{2}\)
(d) 0
Answer
Answer: (b) \(\frac{π}{4}\)
Question 54.
cos-1(\(\frac{√3}{2}\))
(a) \(\frac{5π}{6}\)
(b) \(\frac{π}{6}\)
(c) \(\frac{4π}{9}\)
(d) \(\frac{2π}{3}\)
Answer
Answer: (a) \(\frac{5π}{6}\)
Question 55.
cosec-1(2)
(a) \(\frac{π}{6}\)
(b) \(\frac{2π}{3}\)
(c) \(\frac{5π}{6}\)
(d) 0
Answer
Answer: (a) \(\frac{π}{6}\)
Question 56.
sec-1(2)
(a) \(\frac{π}{6}\)
(b) \(\frac{π}{3}\)
(c) \(\frac{2π}{3}\)
(d) \(\frac{5π}{6}\)
Answer
Answer: (b) \(\frac{π}{3}\)
Question 57.
tan-1(√3)
(a) \(\frac{π}{6}\)
(b) \(\frac{π}{3}\)
(c) \(\frac{2π}{3}\)
(d) \(\frac{5π}{6}\)
Answer
Answer: (b) \(\frac{π}{3}\)
Question 58.
cot-1(-√3)
(a) \(\frac{5π}{6}\)
(b) \(\frac{π}{3}\)
(c) \(\frac{π}{2}\)
(d) \(\frac{π}{4}\)
Answer
Answer: (a) \(\frac{5π}{6}\)
Question 59.
tan-1 + cos-1 (\(\frac{-1}{2}\)) + sin-1 (\(\frac{-1}{2}\))
(a) \(\frac{2π}{3}\)
(b) \(\frac{3π}{4}\)
(c) \(\frac{π}{2}\)
(d) 6π
Answer
Answer: (b) \(\frac{3π}{4}\)
Question 60.
tan-1 (√3) + sec-1 (-2) – cosec-1 (\(\frac{2}{√3}\))
(a) \(\frac{5π}{6}\)
(b) \(\frac{2π}{3}\)
(c) \(\frac{π}{3}\)
(d) 0
Answer
Answer: (d) 0
Question 61.
cos-1 (\(\frac{-1}{2}\)) + 2sin-1 (\(\frac{-1}{2}\))
(a) \(\frac{π}{3}\)
(b) \(\frac{2π}{3}\)
(c) \(\frac{3π}{4}\)
(d) \(\frac{5π}{8}\)
Answer
Answer: (a) \(\frac{π}{3}\)
Question 62.
If cot-1 (\(\sqrt{cosα}\)) – tan-1 (\(\sqrt{cosα}\)) = x then sin x is equal to
(a) tan² (\(\frac{α}{2}\))
(b) cot² (\(\frac{α}{2}\))
(c) tan α
(d) cot (\(\frac{α}{2}\))
Answer
Answer: (a) tan² (\(\frac{α}{2}\))
Question 63.
4 tan-1 \(\frac{1}{5}\) – tan-1 \(\frac{1}{70}\) + tan-1 \(\frac{1}{99}\) is equal to
(a) \(\frac{π}{6}\)
(b) \(\frac{π}{4}\)
(c) \(\frac{π}{3}\)
(d) \(\frac{π}{2}\)
Answer
Answer: (b) \(\frac{π}{4}\)
Question 64.
If 6 sin-1 (x² – 6x + 8.5) = π, then x is equal to
(a) 1
(b) 2
(c) 3
(d) 8
Answer
Answer: (b) 2
Question 65.
Number of solutions of the equation
tan-1 (\(\frac{1}{2x+1}\)) + tan-1 (\(\frac{1}{4x+1}\)) = tan-1 (\(\frac{2}{x^2}\))
(a) 1
(b) 2
(c) 3
(d) 4
Answer
Answer: (b) 2
0 Comments